PPM Advection Scheme¶
Advection Scheme¶
Following [11] and [10], we use the Piecewise Parabolic Method (PPM) to represent values within the model cells. Each cell is assumed to have a piecewise parabolic representation, which is uniquely prescribed by conservation and the two edge values. This method has the following features:
The PPM approach is conservative.
The (unlimited) order of accuracy is determined by the estimates of the edge values.
Monotonicity is ensured by adjusting the edge values to flatten the profile.
An example is shown in this figure:
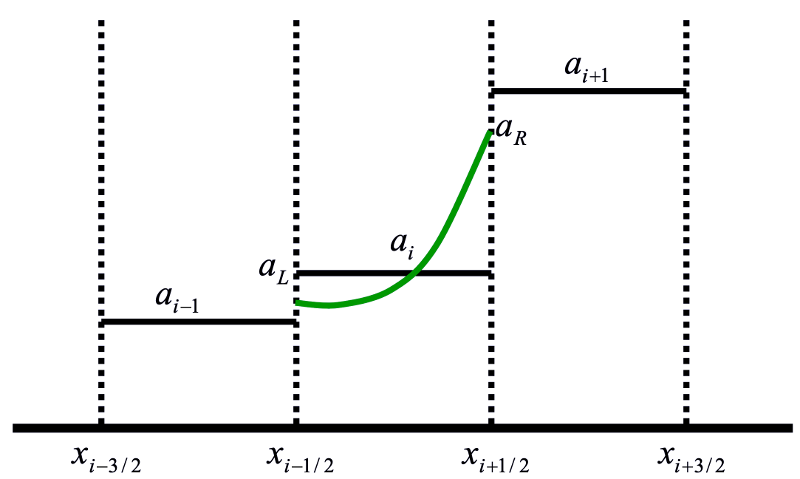
The parabolic representation of a field within a cell.¶
\[x'_i \equiv \frac{x - x_{i-1/2}} {\Delta x_i}\]
\[\Delta x_i \equiv x_{i + 1/2} - x_{i- 1/2}\]
\[c \equiv u \Delta t / \Delta x_i\]
\[A_i(x') = a_L + (a_R - a_L) x'_i + a_6 x'_i(1 - x'_i)\]
\[a_6 = 6a_i - 3 (a_R + a_L)\]
\[\begin{split}\begin{eqnarray} a_i &= \int_0^1 A_i(x'_i) dx'_i = \int_0^1 a_L + (a_R - a_L) x'_i + a_6 x'_i (1 - x'_i) dx'_i \\ &= \left[ a_L x'_i + \frac{1}{2} (a_R - a_L) x_i^{\prime 2} + a_6 \left( \frac{1}{2} x_i^{\prime 2} - \frac{1}{3} x_i^{\prime 3} \right) \right]_0^1 \\ &= \frac{1}{2} (a_R + a_L) + \frac{1}{6} a_6 \end{eqnarray}\end{split}\]
\[\begin{split}\begin{eqnarray} F_{i+1/2} &= \frac{1}{\Delta t} \int_{x_{i + 1/2} - u \Delta t}^{x_{i + 1/2}} A_i^n(x) dx = \frac{\Delta x}{\Delta t} \int_{1-c}^1 A_i (x'_i) dx'_i \\ &= \frac{\Delta x}{\Delta t} \left[ a_L x'_i + \frac{1}{2} (a_R - a_L) x_i^{\prime 2} + a_6 \left( \frac{1}{2} x_i^{\prime 2} - \frac{1}{3} x_i^{\prime 3} \right) \right]_{1 - c}^1 \\ &= \frac{\Delta x}{\Delta t} \left[ a_L c + (a_R - a_L + a_6) \left( c - \frac{1}{2} c^2 \right) - a_6 \left( c - c^2 + \frac{1}{3} c^3 \right) \right] \\ &= u \left[ a_R + \frac{1}{2} (a_L - a_R) c + a_6 \left( \frac{1}{2} c - \frac{1}{3} c^2 \right) \right] \end{eqnarray}\end{split}\]
The choice of \(a_L\) and \(a_R\) is not unique, but can be done according to [11] (CW84) or [34] (H3) as mentioned in Tracer Advection.